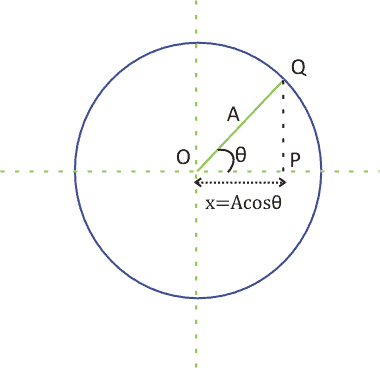
A circle of reference is a graphical representation that facilitates the understanding of various SHM relationships. Consider the figure given below in which a point Q moves anti-clockwise around a circle of radius A with a constant angular velocity ω (in rad.s-1).
In this blog, many times I had written short notes or questions related to the concept of SHM and this article is about yet another important concept of SHM that is Circle of Reference.
Here in the figure
OQ→position of Q relative to O
θ→angle OQ vector makes with the positive x-axis
This vector whose horizontal component represents the actual motion is called a
phasor
Let P be the point representing the projection of point Q (known as the reference point) on the horizontal diameter of the circle (known as the reference circle). As the reference point revolves, the point P moves back and forth along the horizontal diameter always keeping below or above the point Q. The motion of this point P is comparable to that of a body moving under the influence of elastic restoring force and executing SHM in the absence of frictional forces.
From the figure given above, displacement of point P at any given time t is the distance OP or x, and from the figure
x=Acosθ
The angular velocity of circular motion is
ω = angle swept time-taken = θ t
so,
θ=ωt
and
x=Acosωt (1)
The figure given below shows the velocity in SHM using the circle of reference.
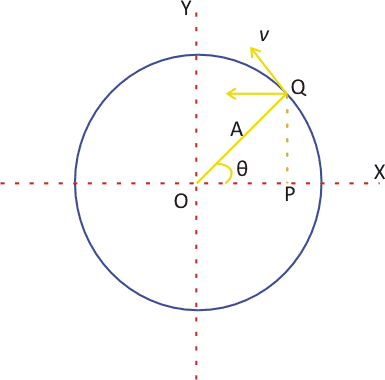
The velocity of the reference point Q would be the component of Q’s velocity parallel to the x-axis since P is always directed below or above the reference point. So,
vP=-vsinθ = -ωAsinωt (2)
since v=ωA is the tangential velocity of motion of reference point Q. The negative sign is introduced because the direction of velocity is towards the left. When Q is below horizontal diameter, the velocity of P is towards the right, but since sinθ is negative at such points, the minus sign is still needed.
The figure given below shows the acceleration in SHM using the circle of reference.
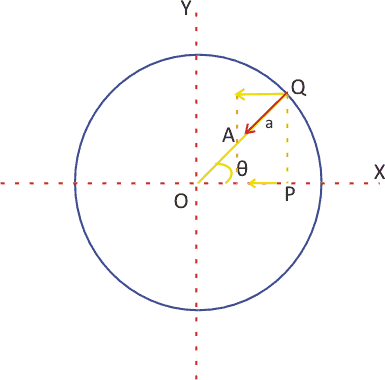
The acceleration of Q on the reference circle is directed radially inwards towards O as can be seen in the figure and its magnitude is equal to
a = v 2 A = ω 2 A
From figure x-component of acceleration is the acceleration of point P. So,
aP=-acosθ= -ω2Acosθ (3)
The minus sign is introduced because the acceleration is towards the left. When Q is to the left of the center, the acceleration of P is towards the right but, since cosθ is negative at such points, the minus sign is still needed.
To prove that motion of point P is simple harmonic, we now use equation 1 in equation 3 then we have
aP=-ω2x
since ω is a constant, the acceleration of point P at any instant is equal to negative constant times displacement xat that instant and this is just the essential feature of SHM and this proves that motion of point P is indeed SIMPLE HARMONIC.
