In this article find the list of current electricity equations or current electricity formulas. Current electricity is related to the flow of electrons. Current electricity is of two types:
- DC or direct current
- AC or alternating current
In this article, we will concern ourselves with electricity equations for direct current. Numericals from this topic are not as tough as mechanics and are relatively easy to solve. Try and remember these formulas; it will help you solve your problems.
Current Electricity Equations
Ohm’s Law :
\[V=IR\]\(V=\) Potential Difference \(I=\) Current \(R=\) Resistance of the conductor
Resistivity or specific resistance:
\[\rho=\frac{AR}{l}\] \(A\) is area of cross-section \(R\) is resistance of conductor and \(l\) is length of the conductor
Resistance of a uniform conductor :
\[R=\rho\frac{l}{A}\]
Conductivity \((\sigma)\) :
\[\sigma=\frac{1}{Resistivity}=\frac{1}{\rho}=\frac{l}{RA}\]
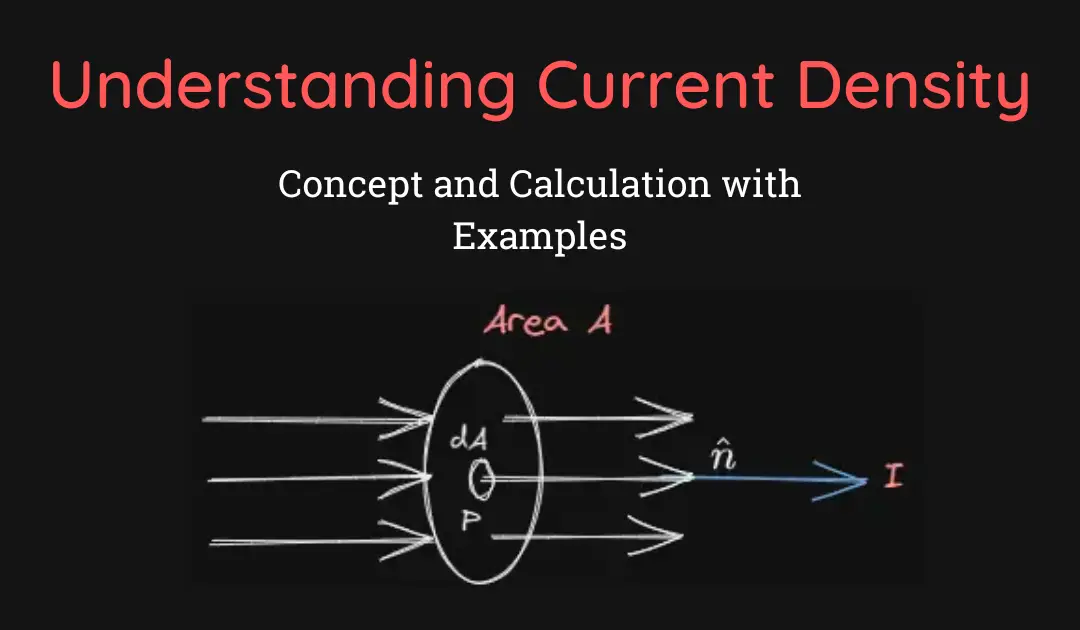
\[j=\frac{Current}{Area}=\frac{I}{A}\]
Current in terms of drift velocity \((v_d)\) :
\[ I=enAv_d\] where \(v_d\) is the drift velocity, \(e\) is the charge on electrons, \(n\) is electron density or the number of electrons per unit volume and \(A\) is the area of cross-section.
Current density:
\[j=env_d\] where symbols have their usual meaning.
Drift Velocity :
\[v_d=\frac{I}{neA}\]
Avogadro Number :
\[N=6.023\times 10^{23}\] Avogadro Number is the number of atoms in one gram atomic mass of an element.
The relation between current density and electric field :
\[j=\sigma E \qquad or \qquad E=\rho j\]
learn more about electric field magnitude
Mobility of charge carriers :
It is the drift velocity acquired by a charge carrier in a unit electric field.
\[\mu=\frac{v_d}{E}\]
Electric current in terms of mobility :
\[I=neAv_d=neA\mu E\]
Temperature coefficient of resistance :
Temperature coefficient of resistance \[\alpha=\frac{R_2 – R_1}{R_1(t_2 -t_1)}\] If \(t_1=0^0C\) and \(t_2=t^0C\) then\[\alpha=\frac{\left (\rho_t-\rho_{0}\right)}{t}=\frac{\left (R_t-R_{0}\right)}{t}\] or, \[R=R_0(1+\alpha t)\]
Resistance in series :
\[R=R_1+R_2+R_3+…….\]
Resistance in parallel :
\[R = \frac{1}{R_1}+\frac{1}{R_2}+\frac{1}{R_3}+……\]
Emf of a cell :
\[\varepsilon=\frac{W}{q}\]Emf is work done by a cell in carrying unit charge along a closed circuit.
EMF for a cell of internal resistance \(r\)
\[\varepsilon=V+Ir=I(R+r)\]
A terminal potential difference of a cell :
\[V=IR=\frac{\varepsilon R}{R+r}\]
Internal resistance of a cell :
\[ r=R\left [ \frac{\varepsilon -V}{V} \right ]\]
Current when n cells are connected in series :
\[I=\frac{n\varepsilon}{(R+nr)}\]
Current when n cells are connected in parallel :
\[I=\frac{n\varepsilon}{(nR+r)}\]
Heat produced by electric current :
\[H=I^2Rt\,\, Joule = \frac{I^2Rt}{4.18}cal\]
\[ H=VIt\,\,Joule=\frac{VIt}{4.18}cal\]
Electric Power :
\[P=\frac{W}{t}=VI=I^2R=\frac{V^2}{R}\]
Electric Energy :
\[W=Pt=VIt=I^2Rt\]
Try this Circuit Symbols Quiz and check how well you know electric circuit symbols.