In physics, we come across quantities that vary over a wide range. For example, we deal with both massive planetary objects like planets and galaxies, as well as very microscopic particles like the nucleus of an atom.
No matter how massive or small a physical quantity is, we need magnitude to describe it.
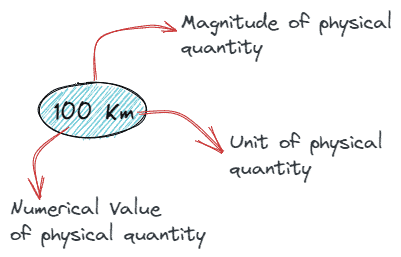
For a physical quantity, magnitude means its numerical value and the units in which it is expressed.
For example, if we say Rohan travelled a distance of 100 Km, then 100 is the numerical value of the physical quantity distance, and Km is the unit of measurement for distance.
In this case, 100 km is a short distance compared to much greater distances between the sun and the earth.
These huge distances are difficult to remember. Instead of memorizing the proper distance, we need to have an approximate idea of the size of the quantity in question.
What is Order of magnitude?
The order of magnitude gives us a rough estimate of the size of a physical quantity, regardless of how large or small its magnitude is. It describes roughly how big a number to the power of 10 is. So, to express such a broad range of numbers, we employ the power of the ten approaches.
If two numbers differ by one order of magnitude, one is roughly ten times greater. They differ by a factor of around 100 if they differ by two orders of magnitude.
Definition
The order of magnitude of a physical quantity is that power of 10 which is closest to its magnitude.
Calculate the order of magnitude.
Let us look at the order of magnitude formula that can be used to find the order of magnitude of a number.
Formula
The order of magnitude of a number $N$ can be expressed as relation $$N=n\times 10^x$$
If $0.5< n\leq5$, then $x$ will be the order of magnitude of $N$.
This condition requires us to convert our number $N$ to a decimal (in a format of $n\times 10^x$) so that our number $n$ in decimals falls between $0.5$ and $5$.
After converting our number to the power of 10 i.e., $x$ gives the order of magnitude of that number.
So, from this condition, we have two simple rules for finding the order of magnitude for calculating the order of magnitude.
How to find order of magnitude
Rule 1:- If we have the first digit of our number between 1 and 4 then decimal comes after the first digit.
Example 1:- Find the order of magnitude of number 2134
We can write this number in the form of $N=n10^x$ which is
$2134=2.134\times 10^3$
here, $x=+3$
so, 3 is the order of magnitude of the number 2134.
Example 2:- Find the order of magnitude of number 0.003548.
We can write this number in the form of $N=n10^x$ which is
$0.003548=3.584\times 10^{-3}$
here, $x=-3$
so, $-3$ is the order of magnitude of number 0.003548.
Rule 2:- If we have the first digit of our number between 5 and 9 then the decimal comes before the first digit.
Example 3:- Find the order of magnitude of number 6678.
We can write this number in the form of $N=n10^x$ that is
$6678=.6678\times 10^4$
here, $x=+4$
so, $+4$ is the order of magnitude of the number 6678.
Example 4:- Find the order of magnitude of number 0.005348.
We can write this number in the form of $N=n10^x$ that is
$0.005348=0.5348\times 10^{-2}$
here, $x=-2$
so, $-2$ is the order of magnitude of the number 0.005348.
Solved questions
Question 1
What is the typical order of magnitude of the base current for a BJT?
(a) $10 ^{-8}$
(b) $10 ^{-9}$
(c) $10^{-6}$
(d) $10^{-3}$
Solution:-
The correct answer is (c) $10^{-6}$
Because the base current is much smaller than the collector and emitter currents, it is normally measured in microamperes.
Question 2
What is the order of magnitude of the seconds present in a day?
Solution:-
We know that
1 day = 24 hours
1 hour = 60 min
1 min = 60 sec
Number of seconds in a day are
$1\,\, day = 24 \times 60 \times 60 = 86400\,\, sec$
In terms of $N=n\times 10^x$
1 day $=0.864\times 10^5 sec$
Hence, the order of magnitude is 5.
Question 3
Find the order of magnitude of a light year.
Solution
One light year is defined as the distance covered by light in one year.
We know that speed of light in vacuum is
$c=3\times 10^8 m/s$
Thus,
1 light year $=3\times 10^8 m/s \times 1\, year$
$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,= 3\times 10^8 m/s \times (365\times 24 \times 3600\, sec)=9.5 \times 10^{15}m$
According to rule 2 of order of magnitude $9.5>5$.
In terms of $N=n\times 10^x$
1 light year $=.95 \times 10^{16}$
Thus, order of magnitude of one light year is $16$.