In this article, we will learn how to calculate distance from acceleration and velocity. We would consider a special case of motion where our object under consideration is moving with constant acceleration.
Here in this article, we will only discuss the formula for calculating the distance from acceleration and velocity only. The formula we would be using does not involve time. However, if you are aware of initial and final velocity and acceleration you can find time using the first equation of motion which is $v=u+at$.
Before going any further we must be aware of all the terms used. So,
Distance:- Distance covered by a moving object refers to how much ground the object has covered without any regard to the direction of motion. SI unit for measuring distance is a meter.
Velocity:- Distance traveled by the moving body per unit of time gives the measure of the velocity of the object. It tells about how far an object moves in a given interval of time. SI unit for measuring velocity is meter per second (m/s).
Acceleration:- Acceleration is the rate of change of velocity of an object with respect to time. Like velocity, it is a vector quantity having both magnitude and acceleration. Its unit is $m/s^2$
Calculate Distance from Acceleration and Velocity
Since we are considering the motion of the body or object with constant acceleration we have an equation of motion that relates all these quantities. This equation is the third equation of motion and is given by the relation
$v^2=u^2+2as$
Here,
$s$ – is the distance traveled by the object in time $t$
$u$ – is the initial velocity
$v$ – is the final velocity and
$a$ – is the constant acceleration of the moving object
You can use this formula in various situations involving distance, initial velocity, final velocity and acceleration motion. You need to have a knowledge of three quantities to find the fourth quantity. It must be noted that this equation does not involve the time interval of motion of the object.
Solved Example
This solved example shows How to Calculate Time and Distance from Acceleration and Velocity
Example 1
A body is thrown up with a velocity of 78.4 m/s. Find how high it will rise and how much time it will take to return to its point of projection?
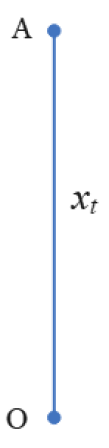
Solution
Let $O$ be the point of projection as shown in the figure.
It is given in the question that at time $t=0$
Initial velocity $u=78.4m/s$ and
$x_0=0$
Let $t$ be the time taken to reach the maximum height. It must be noted that velocity at maximum height is zero. So,
Final velocity $v=0$
According to the question we have to find the distance $x_t$ at time $t$ and time $2t$ which is the time it will take to return to its point of projection.
Here in solving this question, we will use initial conditions and required equations of motion to find variables of motion asked in the question.
The body thrown upwards moves under the effect of the gravitational force of Earth. So, here we will use standard gravity,
$g = -9.8 m/s^2$ ,
for equations involving the Earth’s gravitational force as the acceleration rate of the object.
Here we have taken acceleration due to gravity as negative because we have taken upward direction as positive.
Now,
$v^2-u^2=2g[x_t-x_0]$
putting in all the values we get
$0^2-(78.4)^2=2(-9.8)[x_t-0]$
Therefore,
$x_t=\frac{78.4\times 78.4}{2\times 9.8}m=313.6\,\, m$
Again from the first equation of motion which relates final velocity, initial velocity, acceleration, and time we get
$v=u+at$
plugging in required values we get
$0=7.4+(-9.8)t$
or,
$9.8t=78.4$
0r,
$t=\frac{78.4}{9.8}s=8s$
Since the time of ascent is equal to the time of descent. So total time is taken,
$2t=16s$
Example 2
The reaction time for an automobile driver is 0.7 seconds. If the automobile can decelerate at $5m/s^2$, calculate the total distance traveled in coming to stop from an initial velocity of $30 Km/h$ after a signal is observed.
Note:-
☛ After reading this question you can note that we are given deceleration and the initial velocity, and we have to find the distance traveled in coming to stop.
☛ Here we are also aware that the final velocity of the automobile is zero because the vehicle is coming to stop after the application of the brake.
☛ Here in this question, we have to find the distance in two parts
1. Distance traveled during the response time of 0.7 seconds.
2. Distance traveled while the automobile decelerates.
Solution
Since the reaction time of the driver is $0.4s$ therefore the automobile, during this time, will continue to move with uniform velocity of $30 Km/h$. Since all other units are given in metric system we will convert $30 Km/h$ to $m/s$. So,
$30 Km/h=30\times\frac{1000}{3600} m/s=\frac{25}{3}m/s$
Distance covered during 0.7 second
$=\frac{25}{3}\times 0.7m=5.83m$
Let us choose this time as reference time $(t=0)$ when the automobile begins to decelerate.
So, at
$t=0$,
$x_0=0$,
initial velocity is $u=\frac{25}{3}m/s$ and
acceleration $a=-5m/s^2$.
We have to find the total distance traveled in coming to stop from initial velocity.
Putting these values in third equation of motion $v^2=u^2+2as$ we have
$0^2-\left ( \frac{25}{3} \right )^2=2\times(-5)[x_t-0]$
or,
$x_t=\frac{625}{9}\times \frac{1}{10}m=6.94m$
Total distance travelled $= 5.83 m+6.94 m=12.77 m$
Related Links
- kinematics – How to get distance when acceleration is not constant? – Physics Stack Exchange – An interesting read that discussed how to find distance when acceleration is not constant. It involves calculations using calculus.
- http://hyperphysics.phy-astr.gsu.edu/hbase/index.html – Concept maps of nearly all concepts of physics.
- Units of measurement in metric system