How to find work with mass and distance
How to Find Work with Mass and Distance is an instructional resource that aims to provide guidance on comprehending the principles of physics related to work, specifically in situations where mass and distance are involved.
Introduction to Work in Physics
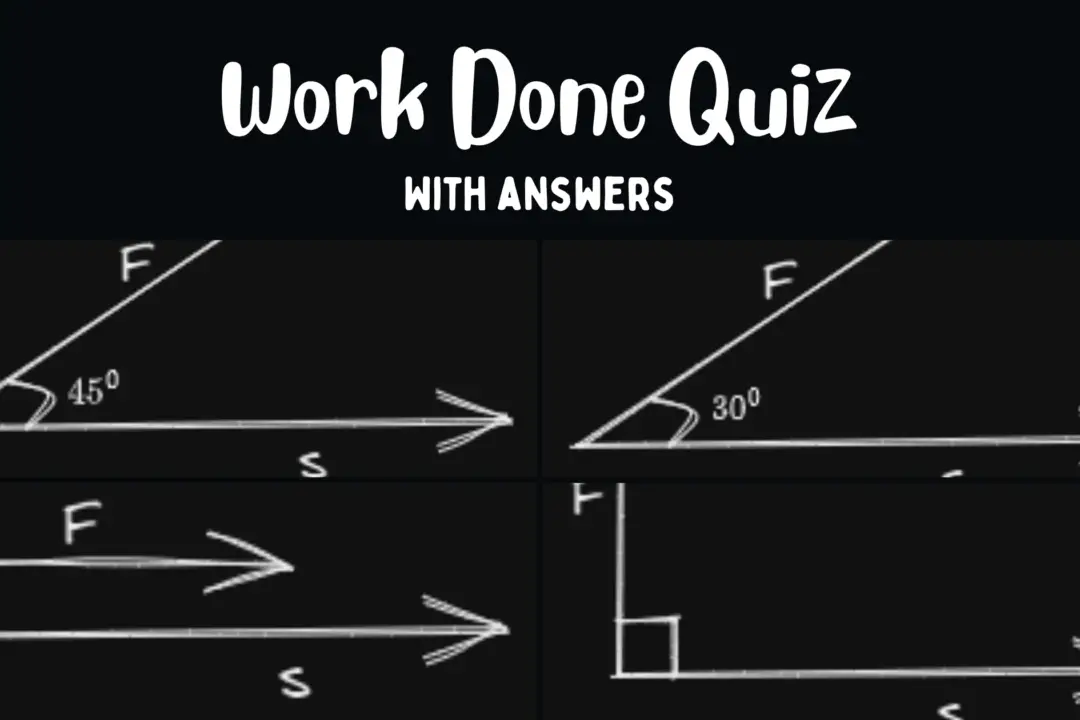
In physics, the concept of work is used to describe the transfer of energy from one system to another. It is a scalar quantity and is defined as the product of the force applied to an object and the distance over which the force is applied in the direction of the force. The SI unit of work is the joule (J).
Mathematical Representation of Work
The formula to calculate work (\( W \)) when a constant force (\( F \)) is applied over a distance (\( d \)) in the direction of the force is given by:
\[
W = F \times d \times \cos(\theta)
\]
Here, \( \theta \) is the angle between the force vector and the direction of motion. If the force is applied in the direction of motion,
\( \theta = 0 \) and \( \cos(0) = 1 \),
simplifying the formula to:
\[
W = F \times d
\]
Work with Mass and Distance
In situations involving mass and distance, the force is often the gravitational force acting on the object. The gravitational force (\( F \)) acting on an object of mass (\( m \)) is given by:
\[
F = m \times g
\]
where \( g \) is the acceleration due to gravity (\( 9.81 \, \text{m/s}^2 \) on Earth).
To find work done in lifting the object to a certain height (\( h \)), you can substitute this force into the work formula:
\[
W = m \times g \times h
\]
Example Calculation
Let’s consider an example where you lift a 5 kg object to a height of 2 meters. The work done would be:
\[
W = 5 \, \text{kg} \times 9.81 \, \text{m/s}^2 \times 2 \, \text{m} = 98.1 \, \text{J}
\]
Related Questions
What is the unit of work?
The SI unit of work is the joule (J).
Is work a vector quantity?
No, work is a scalar quantity.
How is work related to energy?
Work is a form of energy transfer between systems.
What happens if the force is perpendicular to the direction of motion?
If the force is perpendicular to the direction of motion, \( \theta = 90^\circ \) and \( \cos(90) = 0 \), making the work done zero.