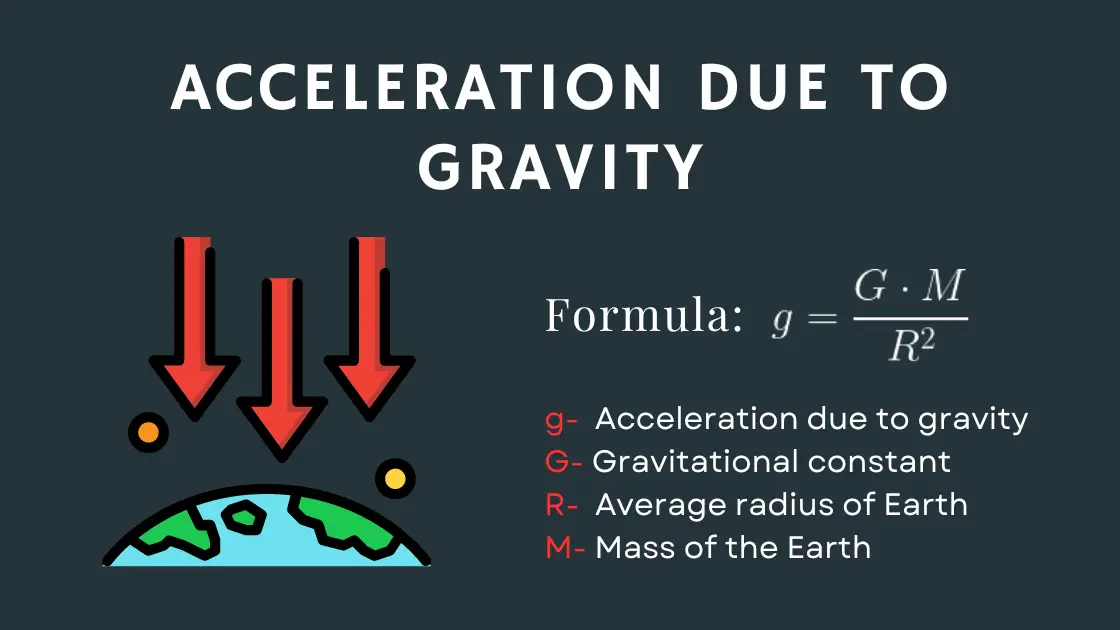
Acceleration due to gravity is a vector quantity. It is used to describe the rate at which an object accelerates towards the center of a massive body, such as a planet. It is the force per unit mass exerted on an object due to the gravitational pull of a celestial body.
Definition of Acceleration Due to Gravity
The acceleration due to gravity (\( g \)) is defined as the acceleration experienced by an object due to the gravitational force acting upon it. Mathematically, it is described by the formula:
\[
g = \frac{{F_{\text{gravity}}}}{m}
\]
Where \( F_{\text{gravity}} \) is the gravitational force acting on the object and \( m \) is its mass.
What is the acceleration due to gravity?
Acceleration Due to Gravity on Earth
On the Earth’s surface, the acceleration due to gravity is approximately \( 9.81 \, \text{m/s}^2 \). This value can vary slightly depending on factors such as altitude and latitude.
The value of $g$ can be calculated using Newton’s Law of Universal Gravitation:
\[
g = \frac{{G \cdot M}}{R^2}
\]
Where,
- \( G \) is the Universal Gravitational Constant \((6.67430 \times 10^{-11} \, \text{m}^3/\text{kg s}^2)\),
- \( M \) is the mass of the Earth \((5.972 \times 10^{24} \, \text{kg})\), and
- \( R \) is the average radius of the Earth \((6.371 \times 10^6 \, \text{m})\).
The constant \( g \) appears in various equations in physics, such as:
- Newton’s Second Law: \( F = m \cdot a \), where \( a \) for objects in free fall near the Earth’s surface is approximately \( g \).
- Gravitational Potential Energy: \( U = m \cdot g \cdot h \)
- Kinematic Equations: \( s = u \cdot t + \frac{1}{2} \cdot g \cdot t^2 \)
While \( g = 9.81 \, \text{m/s}^2 \) is a useful average, it is an approximation. Precise measurements of gravity require accounting for latitude, altitude, and other local factors.
Several techniques exist for measuring \( g \), ranging from simple pendulum experiments to advanced gravimetry techniques that use specialized equipment.
Dimensional Formula of Acceleration Due to Gravity
The dimensional formula of acceleration due to gravity \( g \) can be represented as:
\[
[g] = \text{M}^0 \text{L}^1 \text{T}^{-2}
\]
Acceleration Due to Gravity on the Moon
Basic Value and Comparison with Earth
The acceleration due to gravity (\( g \)) on the Moon is significantly weaker than on Earth. The standard value of \( g \) on the Moon is approximately \( 1.622 \, \text{m/s}^2 \), which is about one-sixth of the Earth’s \( g \) value of \( 9.81 \, \text{m/s}^2 \). This lower value of gravity is one of the reasons why astronauts experience reduced weight and can perform higher jumps on the Moon as compared to Earth.
Factors Influencing the Moon’s Gravity
The Moon’s weaker gravitational pull can be attributed primarily to two factors:
- Reduced Mass: The Moon’s mass is substantially lower than Earth’s. Its mass is approximately \( 7.35 \times 10^{22} \, \text{kg} \), as compared to Earth’s \( 5.97 \times 10^{24} \, \text{kg} \).
- Smaller Radius: The Moon’s radius is also much smaller than that of Earth, approximately \( 1737.5 \, \text{km} \) compared to Earth’s \( 6371 \, \text{km} \).
Mathematical Formulation
The acceleration due to gravity on the Moon can be calculated using the universal formula:
\[
g_{\text{Moon}} = \frac{{G \cdot M_{\text{Moon}}}}{R_{\text{Moon}}^2}
\]
Where \( G \) is the gravitational constant \( 6.67430 \times 10^{-11} \, \text{m}^3/\text{kg s}^2 \), \( M_{\text{Moon}} \) is the Moon’s mass, and \( R_{\text{Moon}} \) is the Moon’s radius.
Impact on Lunar Missions
Understanding the acceleration due to gravity on the Moon is crucial for space missions. The weaker gravity affects everything from the amount of fuel needed for landing and takeoff to the behavior of dust and other particles on the Moon’s surface.
Is the Acceleration Due to Gravity the Same on the Equator and Poles?
No, the value of \( g \) is slightly higher at the poles than at the equator. This is primarily due to the Earth’s oblate spheroid shape and its rotation.
Contrary to a common misconception, the acceleration due to gravity (\( g \)) is not uniform across Earth’s surface. Specifically, the value of \( g \) varies between the equator and the poles. This variability is due to Earth’s shape and its rotational dynamics.
Factors Contributing to the Variation
- Oblate Spheroid Shape: Earth is not a perfect sphere but an oblate spheroid. This means it is slightly flattened at the poles and bulging at the equator. Due to this shape, the distance from Earth’s center to its surface is less at the poles than at the equator.
- Centrifugal Force: Earth’s rotation causes a centrifugal force that pushes objects away from the axis of rotation. This force is maximum at the equator and zero at the poles.
Mathematical Explanation
The effective acceleration due to gravity can be expressed as:
\[
g_{\text{effective}} = g – \omega^2 r
\]
Here, \( \omega \) is the angular velocity of Earth’s rotation, and \( r \) is the radial distance from the axis of rotation to the point of interest (which varies due to Earth’s oblate shape). For the poles, \( r = 0 \), so \( g_{\text{effective}} = g \). At the equator, the term \( \omega^2 r \) is not zero, so \( g_{\text{effective}} \) is slightly less than \( g \).
Measured Values
At the poles, the acceleration due to gravity is approximately \( 9.83 \, \text{m/s}^2 \), whereas at the equator, it is about \( 9.78 \, \text{m/s}^2 \).
Question Answers
Why is the acceleration due to gravity not uniform on Earth?
The Earth is not a perfect sphere; it is an oblate spheroid. This shape, along with variations in Earth’s density, contributes to slight differences in the acceleration due to gravity at different locations.
Why is gravity weaker on the moon?
The moon has both a smaller mass and radius compared to Earth, resulting in a weaker gravitational pull.
Is the acceleration due to gravity constant throughout the Earth’s atmosphere?
No, \( g \) decreases with altitude.
Can the acceleration due to gravity be negative?
The magnitude of \( g \) is always positive, but the direction can be considered negative if opposite to the defined positive direction.
How much does \( g \) vary from the poles to the equator?
At the poles, \( g \) is about \( 9.83 \, \text{m/s}^2 \), and at the equator, it is about \( 9.78 \, \text{m/s}^2 \). The difference is small but significant for precise measurements.
How does the weaker gravity on the Moon affect its ability to hold an atmosphere?
The Moon’s weaker gravity makes it unable to retain a significant atmosphere, which in turn impacts various phenomena like erosion and temperature regulation.