This article contains a list of the center of mass formulas along with some centre of mass problems and examples.
Centre of mass formula list
- For a system of N particles,the position vector of center of mass is \[\vec{R}_{CM}=\frac{m_1\vec{r}_1+m_2\vec{r}_2+………..+m_N\vec{r}_N}{m_1+m_2+…..+m_N}=\frac{\sum_{i=1}^N{m_i\vec{r}_i}}{M}\]
- The position vector of the center of mass of a two-particle system is \[\vec{R}_{CM}=\frac{m_1\vec{r}_1+m_2\vec{r}_2}{m_1+m_2}\]
- The Cartesian coordinates of the center of mass are given by \[X_{CM}=\frac{m_1x_1+m_1x_2+………..+m_Nx_N}{m_1+m_2+…..+m_N}=\frac{\sum_{i=1}^N{m_ix_i}}{M}\] \[Y_{CM}=\frac{m_1y_1+m_1y_2+………..+m_Ny_N}{m_1+m_2+…..+m_N}=\frac{\sum_{i=1}^N{m_iy_i}}{M}\] \[Z_{CM}=\frac{m_1z_1+m_1z_2+………..+m_Nz_N}{m_1+m_2+…..+m_N}=\frac{\sum_{i=1}^N{m_iz_i}}{M}\]
- For Continuous mass distribution \[\vec{R}_{CM}=\frac{1}{M}\int{\vec{r}dm}\]
where dm is the mass element located at position \vec{r}. Also \[X_{CM}=\frac{1}{M}\int{xdm}\] \[Y_{CM}=\frac{1}{M}\int{ydm}\] \[Z_{CM}=\frac{1}{M}\int{zdm}\] - Velocity of center of mass of a two particle system is \[\vec{v}_{CM}=\frac{m_1\vec{v}_1+m_2\vec{v}_2}{m_1+m_2}\]
The motion of the Center of mass examples
A few examples of motion of the center of mass based on the list of the center of mass formulas.
- Binary stars:- Binary stars are two stars bound to each other by gravitational force. Binary stars orbit around their common center of mass.
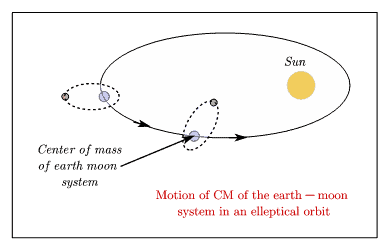
- The Earth-moon system is one such system center of mass of the Earth-Moon system moves around the Sun in an elliptical path, not the earth and moon themselves.
Problems based on Center of mass formulas with solutions
Problem 1
Determine the center of mass of a hemisphere of radius \(R\) and uniform density \(\rho\).
Strategy:
We will divide the given hemisphere into co-axial discs and then find the relation for the center of mass of this disc using the center of mass formula for a continuous distribution of mass. We will integrate this relation for the limits \(0\) to \(R\) for the x-axis. From symmetry \(Y_{CM}\) and \(Z_{CM}\) would be 0.
The mass of the strip can be found using the fact that density is mass per unit volume i.e. \(\rho=m/V\)
Solution
Let \(\rho\) be the density of the material of the hemisphere. Take \(O\) as the origin. The hemisphere can be assumed to be made up of a large number of co-axial discs as shown below in the figure.
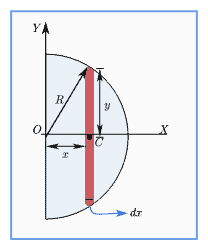
Consider one such elementary disc of radius \(y\) and thickness \(dx\) at a distance \(x\) from the origin.
\(\text{Mass of the elementary disc}= volume \times density\) \[dm=\pi y^2dx\times \rho =\pi \left( R^2-x^2 \right) dx\rho\] The coordinates of the center of mass of the hemisphere can be determined as follows \[X_{CM}=\frac{1}{M}\int{xdm}\] \[=\frac{1}{M}\int_0^R{x\pi \left( R^2-x^2 \right) \rho dx}\] \[=\frac{\pi \rho}{M}\int_0^R{\pi \left( xR^2-x^3 \right) \rho dx}\] \[=\frac{\pi \rho}{M}\left[ R^2\frac{x^2}{2}-\frac{x^4}{4} \right] _{0}^{R}\] Solving and simplifying it we get
\[X_{CM}=\frac{\pi \rho}{M}\left[ \frac{R^4}{4} \right] \] Since, \[M=\frac{2}{3}\pi R^3\times \rho\] for this hemisphere, thus we have \[X_{CM}=\frac{\pi \rho}{\frac{2}{3}\pi R^3\rho}\left[ \frac{R^4}{4} \right] \] Simplifying it we get \[X_{CM}=\frac{3}{8}R\] \(Y_{CM}=0\) and \(Z_{CM}=0\) because of symmetry.
Hence the coordinates of the center of mass of the hemisphere are \[\left( \frac{3}{8}R,\text{0,}0 \right)\]