Distance and displacement questions

In physics, displacement and distance are two crucial concepts. We’ll put your knowledge to the test today with this quiz on displacement and distance. You can test your understanding of distance, and displacement by taking the quiz below. Try it out to see how well you do.

Distance and displacement are the only topics that are used in the questions on this quiz. But students should already know about right-angle triangles and Pythagoras’ theorem, which is used to figure out displacement.

Distance and displacement questions

Question 1
A particle travels along a curved path between two points $P$ and $Q$ as shown:

The displacement of the particle does not depend on

(a) the location of $P$ and $Q$
(b) the choice of origin
(c) the direction of $Q$ from $P$
(d) the length of the line joining $P$ and $Q$

(b)
We know that
Displacement = position vector of final position – position vector of the initial position.
So, it means that displacement depends on the location of $P$ and $Q$ and is independent of the choice of origin.
The direction of displacement is from $P$ to $Q$ and its magnitude is equal to the length of the line joining the final and initial positions.

Question 2
The displacement of a moving object in a given interval of time is zero. Would the distance traveled by the object also be zero?
(a) yes
(b) No
(c) depends on the situation
(d) None of the above

(b)
No, it is not necessary. When a body returns to its starting point after traveling a certain distance, the displacement is zero while the distance traveled is not zero.

Question 3
What is the total displacement of a student who walks 4 blocks east, 2 blocks north, 3 blocks west and then 2 blocks south?
(a) 2 blocks east
(b) 2 blocks west
(c) 1 block west
(d) 1 block east

(d)
The displacement is defined as the shortest distance between two points.

See also  Newton's Law of Motion Multiple Choice Questions (MCQs)

In the given question, a student walks 4 blocks east then walks 2 blocks north, 3 blocks west, and at last 2 blocks South. We can see that the student is one block from where he started. So the starting and ending points are 1 block away from each other towards the east.

Hence, the total displacement of the student is 1 block east.

Question 4
A body moves on three-quarters of a circle of radius $r$. The displacement and distance traveled by it are :
(a) displacement $=r$, distance $=3r$
(b) displacement $=\sqrt{2r}$, distance $=\frac{3\pi r}{2}$
(c) distance $=2r$, displacement $=\frac{3\pi r}{2}$
(d) displacement $=0$, distance $=\frac{3\pi r}{2}$

(b)

From the diagram circumference of the circle $=2\pi r$
three-quarters of a circle of radius $r$
$= \frac{3}{4}\times \text{circumfrance}=\frac{3}{4}\times 2\pi r$
$=\frac{3\pi r}{2}$
Now,
Distance = total path covered by the body
$= \frac{3}{4}\times \text{circumfrance}=\frac{3}{4}\times 2\pi r$
$=\frac{3\pi r}{2}$
Whereas,
Displacement = shortest straight line distance between initial and final position (AB)
$=\sqrt{OA^{2}+OB^2}=\sqrt{r^2+r^2}=\sqrt{2}r$

Question 5
Which of the following is not a vector
(a) displacement
(b) distance
(c) acceleration
(d) velocity

(b)

Question 6
The SI unit of displacement is
(a) meter
(b) centimeter
(c) kilometer
(d) none of these

(a)

Question 7
For any object in motion, distance is always ……………… displacement.
(a) less than or equal to
(b) greater than and equal to
(c) equal to
(d) less than

(b)

Question 8
The motion of a car in the northeast direction for 100 miles tells us about?
(a) distance
(b) displacement
(c) speed
(d) velocity

(b) It is displacement because along with magnitude direction is also mentioned.

Question 9
Distance is a …………. quantity.
(a) scalar
(b) vector
(c) negative
(d) none of these

(a)
Since distance has magnitude and no direction, it is a scalar quantity.

See also  Acceleration time graph

Question 10
Which of the following values can be displacement?
(a) positive
(b) negative
(c) zero
(d) all of these

(d)

Question 11
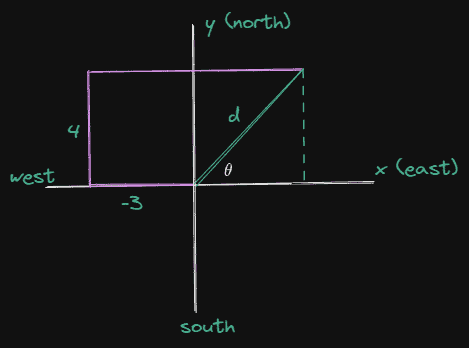
A person moves 30 m north and 20 m towards the east and finally $30\sqrt 2 \, m$ in the south-west direction. The displacement of the person from the origin will be
(a) 10 m along North
(b) 10 m along South
(c) 10 m along West
(d) zero

(c)
Consider the figure given below

It is given in the question that

  • distance moved to north $=30\, m$
  • distance moved to east $= 20\,m$
  • distance moved in south-west direction $=30\sqrt{2}\, m$

In right-angled triangle, $\triangle ECD$ using Pythagoras theorem we get
$EC^2=ED^2+CD^2$
$\Rightarrow \, ED^2=EC^2+CD^2$
$\Rightarrow \, ED^2=(30\sqrt{2})^2-(30)^2$
$\Rightarrow \, ED^2=1800-900$
$\Rightarrow \, ED=30\, m$
Therefore, the displacement of the person from the origin will be
$\Rightarrow \, AE=ED-AD=30-20=10\,m$
The displacement of the person from the origin is 10 m along the west.

Question 12 A particle is moving along the x-axis whose position is varying with time according to relation $x=-3t+t^3$ where $x$ is in meters and $t$ is in seconds. The displacement of particle for $t=1\, s$ to $t=3\,s$ is
(a) $+16m$
(b) $-16m$
(c) $+20m$
(d) $-20m$

(c)
From. $x=-3t+t^3$
at $t=1s$
$x=-3\times 1 +(1)^{3}= -2$
at $t=3s$
$x=-3(-3)+(-3)^{3}=18m$
Required displacement
$x=x_f-x_{i}= 18-(-2)=+20m$

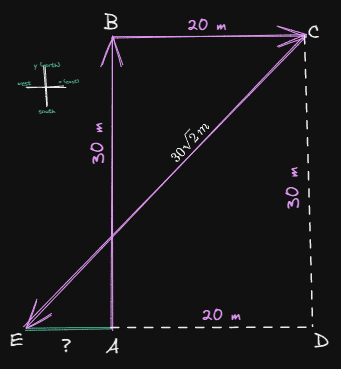
Question 13 A girl delivering newspapers covers her route by traveling 3.00 blocks west, 4.00 blocks north, and then 6.00 blocks east.

(i) What is her resultant displacement? 
(a)$-13.00\;\; blocks$ at $51.1^0$ counterclockwise of East
(b) $-5.00\;\; blocks$ $53.1^0$ clockwise of East
(c) $5.00\;\; blocks$ $53.1^0$ counterclockwise of East
(d) $13.00\;\;blocks$ $51.1^0$ counterclockwise of East

(ii) What is the total distance she travels?
(a)$-13.00\;\; blocks$
(b) $-5.00\;\; blocks$
(c) $5.00\;\; blocks$
(d) $13.00\;\;blocks$

(i) – (c)
Consider the figure given below for the solution

See also  Uniform Acceleration

Her net displacement along x-axis (east-west) $=-3+0+6=+3 \;\;blocls$
Her net displacement along y-axis(north-south) $=0+4+0=+4 \;\; blocks$
The resultant displacement (from the Pythagorean theorem) is
$d = \sqrt{(4^2+ 3^2)} = 5.00\;\; blocks$
the angle the resultant makes with the x-axis (eastward direction) is
$\theta = \tan^{-1}\frac{4}{3}=\tan^{-1}(1.33)=53.1^0$
(ii) – (d)
The total distance traveled is $3.00+4.00+6.00=13.00\;\; blocks$

Question 14
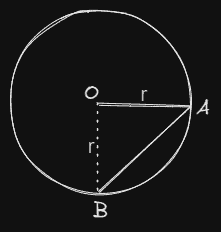
Distance and displacement of a body when it moves half a circle of radius $r$ is
(a) $r,r$
(b) $\pi r, 2r$
(c) $2\pi r, r$
(d) $\pi r,0$

(b)

Question 15
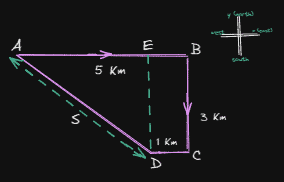
A boat sailing through a river moved eastward for 5 km, then cross the river by moving 3 km southward. On reaching the other side it moved westward through 1 km and reached the jetty. The distance covered and displacement of the boat respectively is
(a) 9 Km, 4 Km
(b) 5 Km, 5 Km
(c) 9 Km, 5 Km
(d) 5 Km, 9 Km

Solution
(c)
From the figure

$AB=5\, Km$
$ED = BC = 3\, Km$
$CD = 1\, Km$
Therefore
$AE=5\, Km – 1\, Km = 4 \,Km$
Distance covered $= AB+BC+CD = 5\, Km+ 3\, Km + 1\, Km = 9\, Km$
Displacement
$S= AD$
$\sqrt{(AE)^2+(ED)^2}=\sqrt{4^2+3^2}=5\,Km$

Important points to remember

  • The smallest distance that can be traveled between two positions determines the magnitude of the displacement. $distance\geq displacement$
  • There can never be zero distance for a moving particle. However, a particle’s displacement can be zero, positive, or negative.
  • Zero displacements indicate that, after motion, the particle has returned to its starting position.
  • While displacement can decrease over time for a moving object, distance can never do so.
  • When displacement lessens over time, the body is returning to its starting position.

Related Posts: