How to find displacement on a velocity-time graph

Let us learn How to find displacement on a velocity-time graph. Finding displacement on a velocity-time graph is extremely easy. The area under a velocity-time graph gives the measurement of the displacement of the object under consideration. A velocity-time graph is a graph between the time taken and the velocity acquired by the moving object during that time.

Here time taken is plotted along the x-axis and velocity acquired is plotted along the y-axis we know that displacement of an object is the product of velocity and time i.e.,\(d=v\times t\)

In this article, we will explore how displacement can be calculated using the velocity-time graph. Let us look at the following cases

Case I: When velocity is uniform (or constant)

To understand this, consider a truck is moving along a straight line with a uniform velocity of 50 Km/hr. The velocity-time graph for this man moving with uniform velocity is

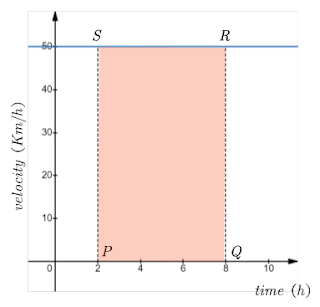
From the above figure, we can see that graph for truck moving with a uniform velocity of 50 Km/h is s straight line parallel to the time axis.
Displacement covered by this truck in between time \(t_1=2 h\) at \(P\) to \(t_2=8 h\) at \(Q\) is given by
Displacement \(d=50 \times (t_2-t_1)\)
Or, \(d=50 \times (8-2)=50 \times 6 = 300 Km\)

From the above graph, we can clearly see that

\(PS=50 Km/h\) and

\(PQ=t_2-t_1=6h\)

Hence Displacement \(=PS \times PQ = \text{Area of rectangle PQRS}\) as shown in the shaded region.

Case II: When acceleration is uniform or constant

For this case let us consider the table given below which shows the change in velocity of a truck at regular intervals of time.

See also Instantaneous velocity

Time in (s)	Velocity of truck (m/s)
0	0
10	4
20	8
30	12
40	16
50	20
60	24

The table given above shows the velocity-time relationship of the truck. We get the following graph when we plot this information graphically

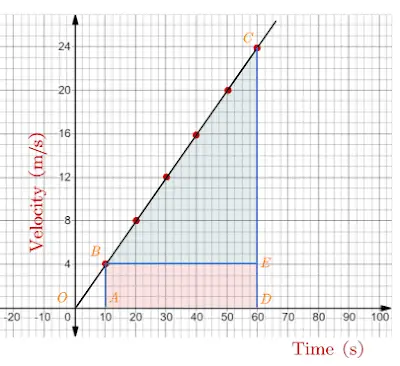
From the graph, we can see that it is a straight line which shows velocity is increasing by equal amounts in equal intervals of time. So, this motion represents a motion with uniform acceleration.The area under this line OC on a velocity-time graph is equal to the displacement of the truck (or object under consideration).

In this case, the displacement covered in any time interval can be found by drawing the perpendiculars on the time axis (for example AB and DC showed in the above figure) at given times. The area between these perpendiculars under the graph gives the displacement.

Let us now find the distance covered by truck between time intervals 10 s and 60 s.
\begin{align*} \text{Distance covered } &= \text{Area of trapezium ABCDA} \\ &=\frac{AB+DC}{2}\times AD \\ &=\frac{4+24}{2} m/s \times (60-10)s \\ &= 14 m/s \times 50 s \\ &= 700 m \end{align*}
Alternatively, for finding displacement from this v-t graph, we can break the shape of the graph into simple geometric shapes. The total area under the line can be calculated by adding the areas of those shapes.
For example, here for finding displacement of the truck between time intervals 10s and 60s we can break this graph into two shapes
1. Triangle BCE and
2. Rectangle ABED
We can then find the areas of both these shapes and add them to get the net displacement between given time intervals.

Case III: When velocity and acceleration both are variable or non-uniform

The figure given below shows the velocity-time graph of the body moving with variable velocity.

v-t graph for non uniform velocity or acceleration

For such cases, we will divide time interval \(t_1\) and \(t_2\) into small intervals of time \(\Delta t\), as there would be a negligible change in velocity during this time interval \(\Delta t\) and hence velocity can be taken as constant.

Therefore for this small time interval displacement \(\Delta s = v\Delta t\) or area of the red strip.

For a complete time interval between \(t_1\) and \(t_2\) displacement would be equal to the area enclosed between the curve and time axis between \(t_1\) and \(t_2\) or the area of the portion \(PQRS\).

Is area under velocity-time graph distance or displacement
It is particularly important to note that