In this article, we will learn about various units for acceleration. Acceleration is a vector quantity that describes the rate of change of velocity of an object over time. In simpler terms, it tells us how quickly an object is speeding up or slowing down.
Learning and understanding different units of acceleration is essential for solving problems in physics, particularly mechanics.
What is acceleration?
Mathematically, acceleration (\(a\)) is defined as the change in velocity (\( \Delta v \)) per unit time (\( \Delta t \)):
\[
a = \frac{\Delta v}{\Delta t}
\]
Units for acceleration
In this section, we will explore the units of acceleration across various systems of units, including the International System (SI), Imperial, and specialized units. Understanding these units is crucial for accurate measurements and calculations in physics and engineering.
SI Unit of Acceleration
- In the International System of Units (SI), the standard unit for acceleration is \( \text{m/s}^2 \) (meters per second squared). This unit is derived from the formula for acceleration:\[
a = \frac{\Delta v}{\Delta t}
\] - Here, \( \Delta v \) is the change in velocity measured in meters per second (m/s), and \( \Delta t \) is the change in time measured in seconds (s).
Conversion Factors between SI unit of acceleration other units of acceleration
1. \( 1 \, \text{g} = 9.81 \, \text{m/s}^2 \)
2. \( 1 \, \text{ft/s}^2 \approx 0.3048 \, \text{m/s}^2 \)
3. \( 1 \, \text{in/s}^2 \approx 0.0254 \, \text{m/s}^2 \)
4. \(1 \text{ m/s}^2 = 100 \text{ cm/s}^2 \)
CGS Unit of Acceleration
- In the Centimetre-Gram-Second (CGS) system, the unit of acceleration is \( \text{cm/s}^2 \) (centimeters per second squared).
- Similar to the SI unit, it is derived from the formula for acceleration but uses centimeters and seconds as the base units.
Imperial Unit of Acceleration
- In the Imperial system, commonly used in the United States, the unit of acceleration is \( \text{ft/s}^2 \) (feet per second squared).
- This unit is also derived from the formula for acceleration, using feet and seconds as the base units.
- $in/s^2$ (inches per second squared) is less unit common but is still used in some engineering contexts.
Astronomical Unit of Acceleration
- In astronomical contexts, acceleration can be measured in \( \text{AU/year}^2 \) (astronomical units per year squared).
- This unit is used to describe the acceleration of celestial bodies over large distances.
Natural Unit of Acceleration
- In certain specialized contexts, such as in theories of relativity, the unit \( \text{c/year} \) (speed of light per year) can be used to describe acceleration at relativistic speeds.
Non-Standard Unit of Acceleration
- In some cases, acceleration is measured in \( \text{g} \) (g-force), especially when describing gravitational or mechanical forces.
- One \( \text{g} \) is approximately \( 9.81 \, \text{m/s}^2 \).
Understanding the acceleration units in these various systems enables more exact and context-appropriate measurements and calculations.
Table of Conversion Factors for Units of Acceleration with Respect to SI Unit \( \text{m/s}^2 \)
Certainly, a table for conversion factors can be a useful reference for quickly converting between different units of acceleration with respect to the SI unit $ \text{m/s}^2 $. Here’s how you can convert various units of acceleration to $ \text{m/s}^2 $:
| Unit of Acceleration | Conversion Factor to $ \text{m/s}^2 $ | Formula for Conversion |
|---|---|---|
| $ \text{m/s}^2 $ (SI Unit) | 1 | $a_{\text{m/s}^2} = a_{\text{m/s}^2}$ |
| $ \text{cm/s}^2 $ (CGS Unit) | $ 1 \times 10^{-2} $ | $ a_{\text{m/s}^2} = a_{\text{cm/s}^2} \times 10^{-2} $ |
| $ \text{ft/s}^2 $ (Imperial Unit) | $ \approx 0.3048 $ | $ a_{\text{m/s}^2} = a_{\text{ft/s}^2} \times 0.3048 $ |
| $ \text{AU/year}^2 $ (Astronomical Unit) | $ \approx 1.503 \times 10^{-11} $ | $ a_{\text{m/s}^2} = a_{\text{AU/year}^2} \times 1.503 \times 10^{-11} $ |
| $ \text{c/year} $ (Natural Unit) | $ \approx 9.454 \times 10^{-9} $ | $ a_{\text{m/s}^2} = a_{\text{c/year}} \times 9.454 \times 10^{-9} $ |
| $ \text{g} $ (Non-Standard Unit) | $ 9.81 $ | $ a_{\text{m/s}^2} = a_{\text{g}} \times 9.81 $ |
Note: The conversion factors are approximate and rounded for simplicity. Always use the most accurate values for precise calculations.
Questions and Answers
What is the acceleration if the velocity of an object changes from 10 m/s to 20 m/s in 2 seconds?
Using the formula
\( a = \frac{\Delta v}{\Delta t} \),
\( a = \frac{20 \text{ m/s} – 10 \text{ m/s}}{2 \text{ s}} = 5 \text{ m/s}^2 \)
How do you convert an acceleration value from \( \text{m/s}^2 \) to \( \text{ft/s}^2 \)?
To convert from \( \text{m/s}^2 \) to \( \text{ft/s}^2 \), multiply the value by 3.28084.
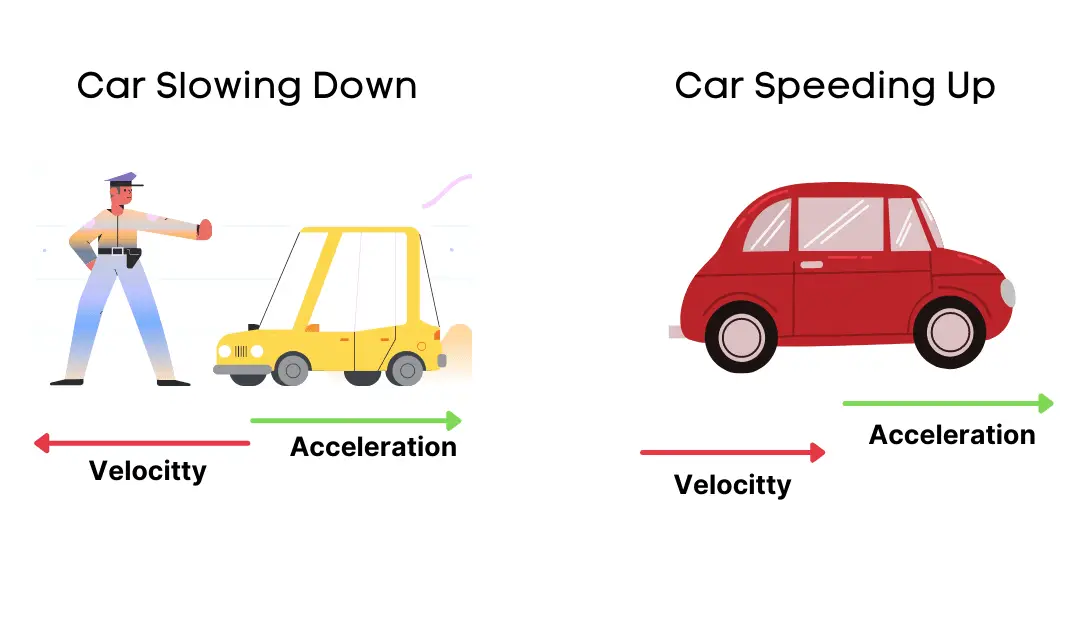
What is negative acceleration?
Negative acceleration, often referred to as deceleration occurs when an object slows down. The units remain \( \text{m/s}^2 \), but the value is negative.
Is acceleration always positive?
No, acceleration can be negative, positive, or zero, depending on whether the object is slowing down, speeding up, or moving at a constant velocity, respectively.
What are the main types of acceleration?
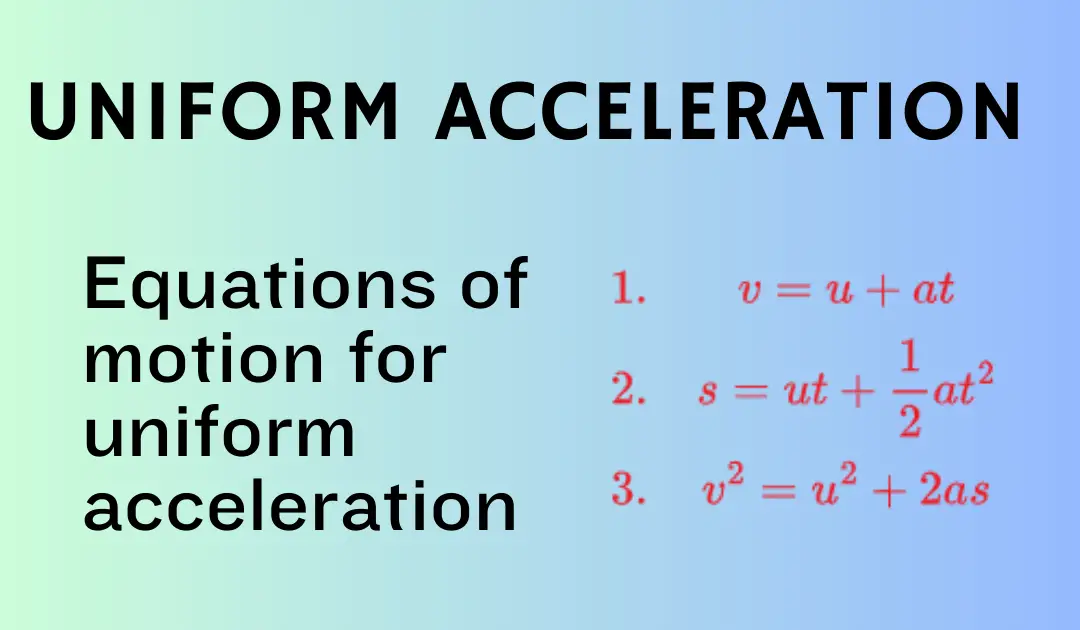
The main types of acceleration are uniform and non-uniform acceleration.
- Uniform acceleration occurs when an object’s velocity changes at a constant rate, such as in free-fall under gravity.
- Non-uniform acceleration happens when the rate of change of velocity varies with time, often seen in complex motions like that of a pendulum.